Section 4: KC Simulation
To demonstrate this power visually, consider the following graphs where two bettors pursue different investment methods while picking the exact same sides of games. Each bettor starts with $100 and wins 55% of his games and gets paid 10:11 odds when he wins. The first bettor (blue line) bets exactly $5.5 per game forever, while the second bettor (red line) utilizes the Kelly criterion and bets 5.505% of his bankroll, decreasing his bet size after losses and increasing it after wins. To create these graphs, we used a random number generator to determine whether a particular game that both bettors were wagering on was a win or a loss.
Through 50 bets
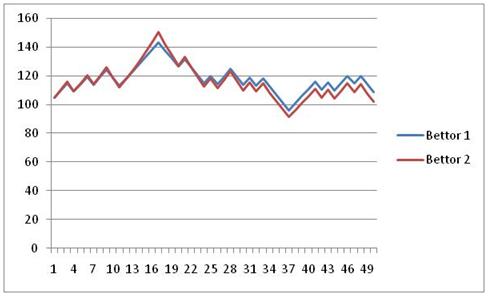
Bettor 1: 108 Bettor 2: 102
In the extremely short term, there is little difference between an investor using the KC and the flat bettor. When a bettors’ short-term record is very good or very bad, the KC will outperform the straight bets, while records near .500 will slightly favor straight betting.
Through 150 bets
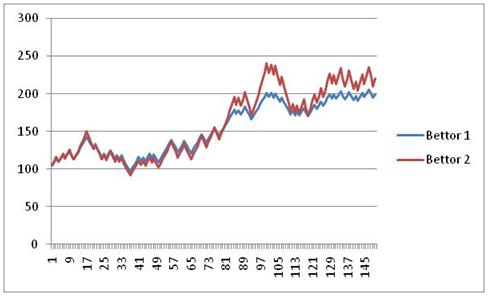
Bettor 1: 199 Bettor 2: 220
Still in the short term, you can see how the KC bets move drastically away from the flat bets on good runs but falls quickly on bad runs. However, as they swing up and down, the KC bets slowly separate themselves.
Through 400 bets
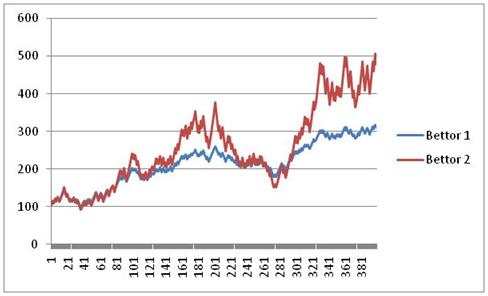
Bettor 1: 316 Bettor 2: 460
As we begin to amass a reasonable sample size, we can see the Kelly bettor beginning to pull away. With a bankroll of $460, the Kelly bettor will wager $25.32 on his next game, while the straight bettor is still betting $5.5 per game.
Through 1000 bets
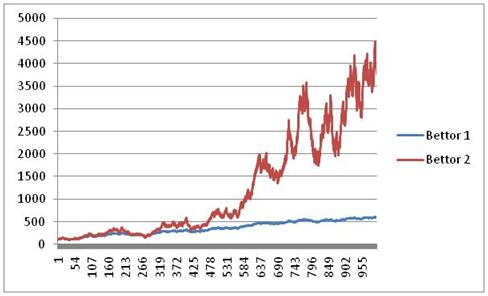
Bettor 1: 608 Bettor 2: 4,148
As we move into 1000 sequential bets, the Kelly bettor with his optimally increasing bet size crushes the straight bettor. At 1000 bets, the straight bettor is still betting the same $5.5 per game that he was at the beginning of the season, while the Kelly bettor will bet $228.34 on his next game. Notice that though the KC bettor is crushing the flat bettor, he also must endure several up and down swings of more than $1000. In fairness, this is also a fairly tame sample, although it was not engineered to be so and rather was just the product of a random number generator. Other samples have even more radical swings than this one, crashing up and down violently in pursuit of the overall upward trend.
Also, keep in mind how stressful these swings of Kelly Betting can be. Around bet #740, the Kelly bettor hits $3500. He then goes on a massive downswing over his next 100 bets (think: an entire football season) and loses half of his bankroll! It’s not until after his 900th bet that he again crosses $3500. Then, over the next 50 or so bets, he swings up to $4000, down to $2800, up to $4500, and so forth. While growth is being optimized by full Kelly wagers, a bettor who cannot keep his eye in the long term may find the swings too unpleasant to handle… especially in a scenario where you don’t know beyond all doubt that you have a long-term edge.
Through 3000 bets
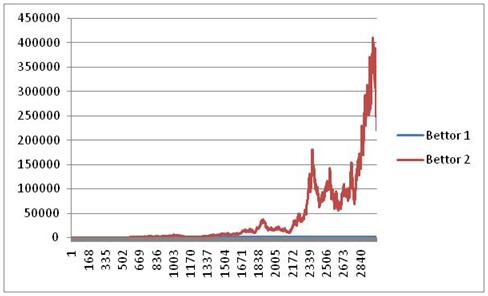
Bettor 1: 1,279 Bettor 2: 219,530
Over 3000 bets, the tremendous advantage of Kelly betting becomes even clearer. The Kelly bettor has run his bankroll to $219,530, while the straight bettor is at only $1,279. Again, notice the drop from $400k to $200k over the last few bets to close out the season.
Through 75000 bets

Bettor 1: 2,571 Bettor 2: 185,749,278
The final example is more of an academic exercise to drive the concept of Kelly betting home – after 7,500 bets the straight bettor has increased his initial $100 to a respectable $2,571… but the Kelly bettor has run his initial $100 to an astounding $185 million. This exercise serves to illustrate the tremendous advantage created by compounding bet sizes to grow one’s bankroll.
In addition, providing for optimal growth, the Kelly Criterion also never allows the bankroll to drop to zero. Even if the bettor experiences terrible luck and loses a long string of games in a row despite being on the correct side, they cannot go broke, as they will have wagered increasingly low amounts as they ride their cold streak. In the long run, any positive expectation wager bet according to the Kelly Criterion will grow to infinity, continually increasing by ever larger amounts -and no matter how bad it runs for a period of time, it can never, ever hit zero.
Those who do not fully understand the nuances and implications of the Kelly Criterion often regard it as either mystical mathematical voodoo beyond the realm of common understanding, or as a misguided gambler’s foolhardy get rich quick scheme. In reality, it is just a valuable and underutilized tool for people who are consistently making positive expectation picks over the very long term and is useful for maximizing expected growth while controlling the volatility to growth ratio. In the presence of uncertain information (i.e. true win percentages), Kelly betting should be tempered or fractionalized. Further explanation, examples and discussion of the Kelly Criterion are provided in the following sections.

